A cell is the smallest unit of life. Cells of different species, organs, and tissues have different morphologies, and morphology characterizes their mechanical properties and functions. What kind of mathematical/physical equations can accurately describe cell morphology and mechanics is an important and fascinating problem for biomechanics, cell biology, and developmental biology.
On Jan. 14, 2022, the labs of Prof. Lei Zhang and Prof. Chao Tang in Peking University published a collaborative research article on PLoS Computational Biology, entitled Computable early Caenorhabditis elegans embryo with a phase field model (link: https://doi.org/10.1371/journal.pcbi.1009755), which constructed a phase field model to accurately compute the morphological evolution of C. elegans embryo in vivo (Figure 1). The model can infer the underlying cellular mechanical properties and was utilized to analyze the C. elegans embryogenesis during 6-, 7-, and 8-cell stages [1].
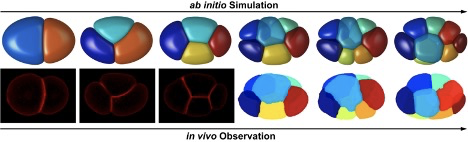
Figure 1. The C. elegans embryonic morphologies from ab initio simulation and in vivo observation.
C. elegans has accurate developmental programs at cellular level, i.e., each cell has reproducible division timing, division orientation, migration trajectory, and identity and fate among individual embryos [2]. Due to the low noise and repeatability of this organism, since 2 decades ago many studies have been carried out to build mechanical models (e.g., multi-particle model and coarse-grained model) to reconstruct its morphological evolution in a computer [3,4]. However, the previous models were usually limited by too many parameters or too low precision, and the details in cell morphology and movement (e.g., cell-cell contact area) could not be calculated fully and accurately [5].
The team constructed a phase field model considering cell surface tension, repulsion and attraction between cells, cell volume constriction, and confinement of the eggshell on cells. The phase field model describes a cell as a constrained diffusible field to characterize its morphology (Figure 2). Then, the team utilized the morphological atlas of C. elegans embryo established before to determine the system parameters and took it as the ground truth for model performance test [6]. With the experimentally measured cell division order, direction, and volume ratio inputted, the predicted embryonic morphologies and motions from 1- to 8-cell stages were well consistent with the experimental observations (Figure 1), and the conserved cell-cell contact map in vivo was completely reproduced. By comparison between the embryo structures in silico and in vivo, the model inferred the substantially weak attraction/adhesion in EMS-P2 contact at 4-cell stage and ABpl-E contact at 8-cell stage, which were verified by two recent experimental reports (Figure 3) [7,8].
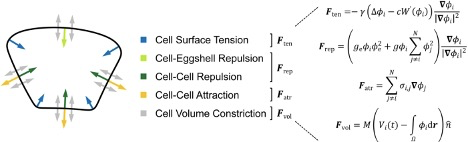
Figure 2. The phase field model that describes cell morphology and mechanics.
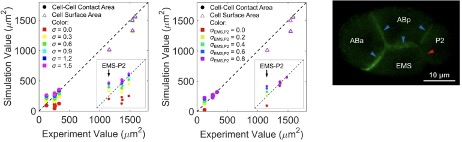
Figure 3. The theoretical prediction of asymmetric cell-cell attraction at 4-cell stage
(σ, global attraction, σEMS, P2, local attraction in EMS-P2 contact).
Left: Linear fitting of global attraction;
Middle: Linear fitting of local attraction in EMS-P2 contact;
Right: Substantially low accumulation of adhesive HMR-1 protein in EMS-P2 contact (indicated by red arrow).
Based on the phase field model, the team next focused on how the cell division orientation, cell division timing, and cell-cell attraction matrix affect the developmental path of embryonic morphology. 1. The cell division orientation programmed in reality, including both the volume segregation direction and ratio, is critical for the stereotypic pattern formations in the real C. elegans embryo. 2. A timely cell division can improve an embryo's robustness against lateral compression and prevents the collapse of both cell-cell contact map and embryo structure; the prediction that an embryo tends to "planarize" under lateral compression was experimentally verified by artificially stopping cell division. 3. The cell-cell attraction provides high diversity for the developmental paths and the experimentally-observed weak attraction in ABpl-E contact at 8-cell is the only single motif/regulation that generates a stable and normal 3D embryo structure. The simulations above show that C. elegans has developed and optimized many genetic programs to ensure its precise and robust embryogenesis.
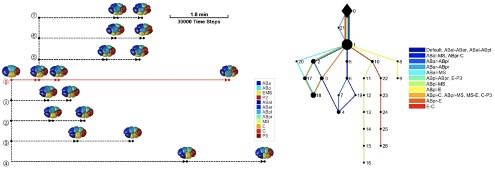
Figure 4. The tree of developmental paths differentiated by cell division timing and cell-cell attraction matrix.
Left: Multiple developmental paths during 6- to 8-cell stages, induced by different cell division timing.
Right: Multiple developmental paths at 8-cell stage, induced by different attraction motif on specific cell-cell contact.
The phase field model proposed in this paper has been verified by experiments carefully. On the one hand, it serves as an effective computational tool for the accurate simulation of cell morphology and mechanics; a solid model can not only help interpret the coding logic of genetic programs in a natural living organism but also be applied to engineering such as the optimization/design of multicellular machines [9]. On the other hand, the simple model successfully calculates the morphological evolution of the real embryo and infers the underlying mechanical information; by finding proper mathematical/physical equations for model construction and avoiding parameter overfitting, one can compute and understand the biological process more comprehensively.
In this paper, Prof. Lei Zhang and Prof. Chao Tang are the corresponding authors; Ph.D students Xiangyu Kuang and Guoye Guan are the co-first authors; Prof. Zhongying Zhao, Dr. Ming-Kin Wong, and Lu-Yan Chan from Hong Kong Baptist University provided experimental supports. This work was supported by the National Natural Science Foundation of China, the National Key R&D Program of China, the Hong Kong Research Grants Council, and the HKBU Interdisciplinary Research Cluster Fund. The team is focusing on the development of methodology for data collection, construction of mathematical/physical models, and discovery of principles and strategies in metazoan embryogenesis.
References:
[1] Kuang & Guan, et al. PLoS Comput. Biol., 2022, 18: e1009755.
[2] Sulston, et al. Dev. Biol., 1983, 100: 64-119.
[3] Kajita, et al. Genome Inform., 2002, 13: 224-232.
[4] Fickentscher et al. Biophys. J., 2013, 105: 1805-1811.
[5] Guan, et al. J. Phys.: Conf. Ser., 2020, 1592: 012020.
[6] Cao & Guan & Ho, et al. Nat. Commun., 2020, 11: 6254.
[7] Yamamoto, et al. Development, 2017, 144: 4437-4449.
[8] Dutta, et al. Front. Cell Dev. Biol., 2019, 7: 209.
[9] Toda, et al. Science, 2018, 361: 156-162.